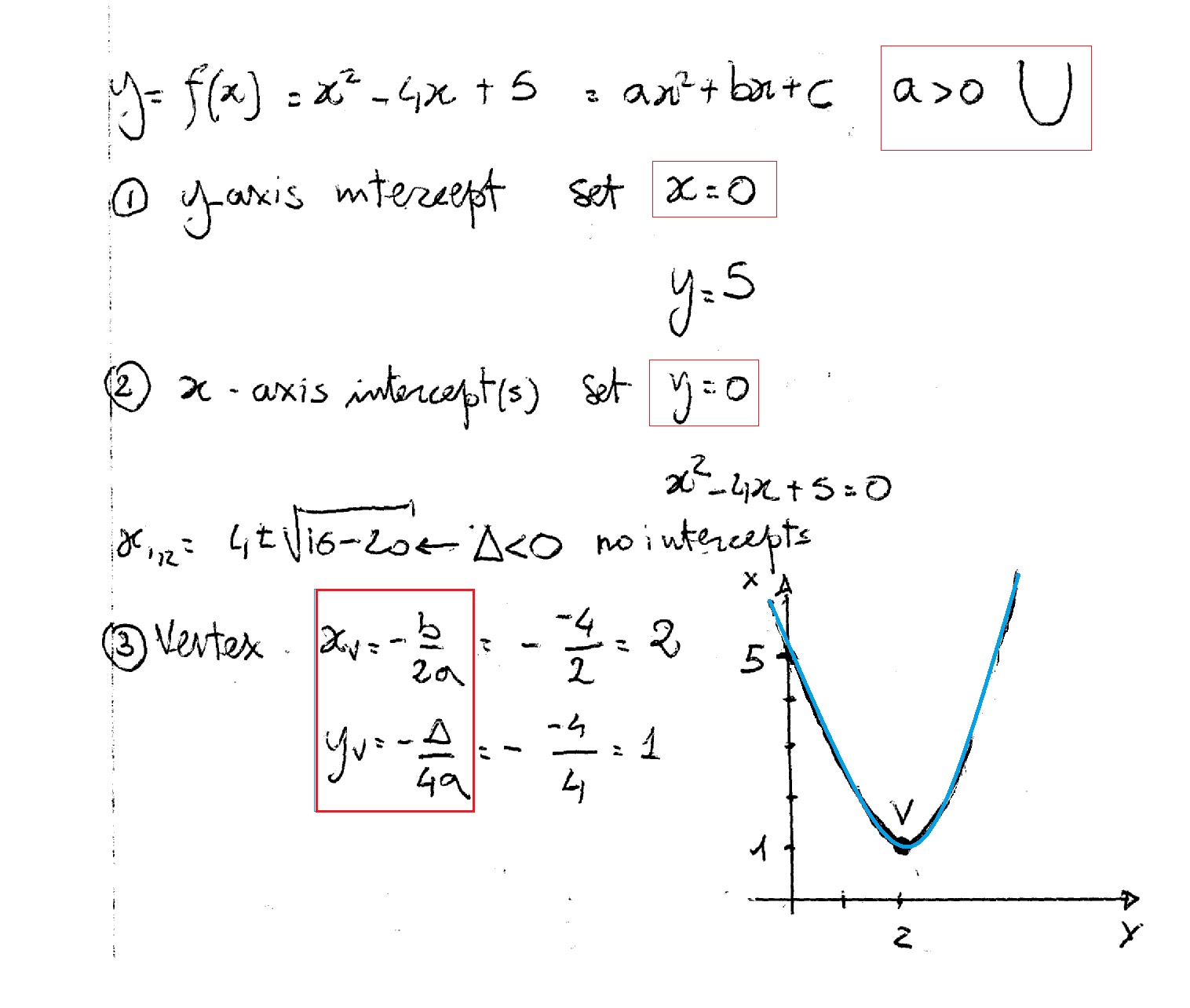
上 y=x^2-4x 4 graph 134115-Y=x^2+4x+1 graph
The Graph For Equation Y X 4 Is Shown Below Which When Graphed With Given Brainly Com Draw The Graphs Of Linear Equations Y X And On Same Cartesian Plane What Do You Observe Sarthaks Econnect Largest Education Community Sonia Graphs The Equations Y X 2 4x And 4 To Solve Equation Her Graph Is Shown BelowFlip the graph over the line y=x to see the inverse This site cannot display it because it is not a function Consider the standard form y = ax2 bx c Write this as y = a(x2 b a x) c Then xvertex = axis of symmetry = ( − 1 2) × b a In this case a = 1 So for y = x2 4x 4 xvertex = ( − 1 2) ×4 = −2 So by substitution for x yvertex = ( −2)2 4( − 2) 4 = 0
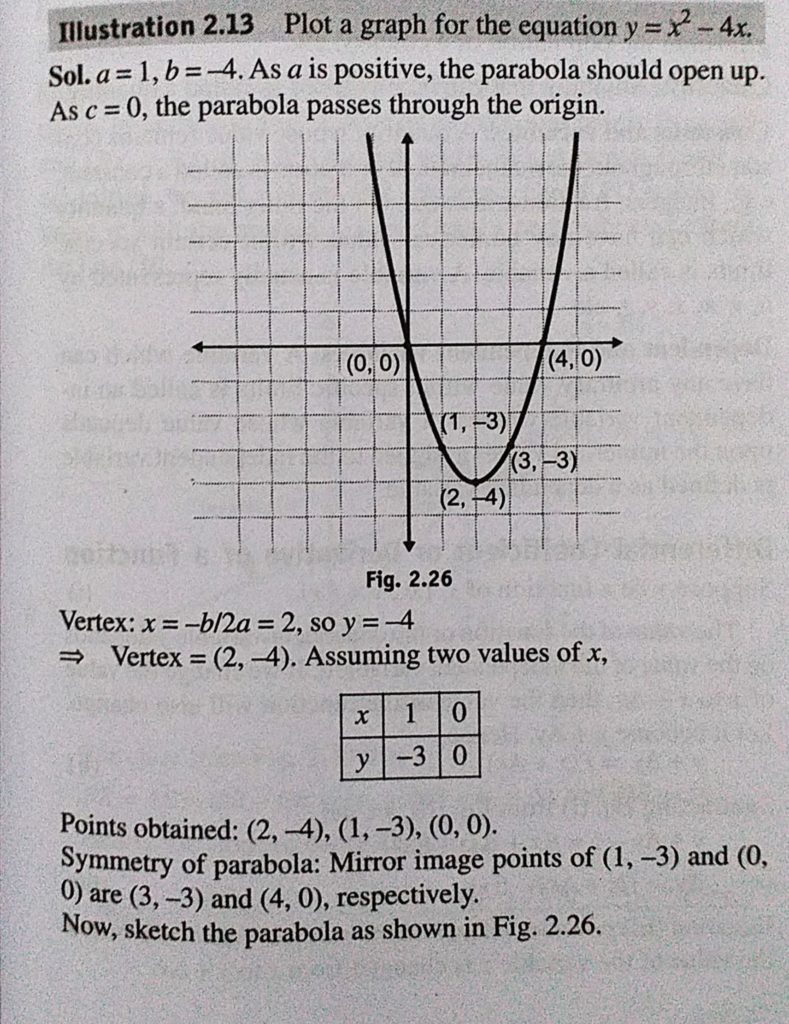
Plot The Graph For The Equation Y X 2 4x Sahay Sir
Y=x^2+4x+1 graph
Y=x^2+4x+1 graph- y = x2 − 4x 4 or y = (x −2)2 0 This is vertex form of equation , y = a(x −h)2 k;Popular Problems Precalculus Graph y^2=4x^2 y2 = 4 − x2 y 2 = 4 x 2 Move −x2 x 2 to the left side of the equation because it contains a variable y2 x2 = 4 y 2 x 2 = 4 This is the form of a circle Use this form to determine the center and radius of the circle (x−h)2 (y−k)2 = r2 ( x h) 2 ( y k) 2 = r 2
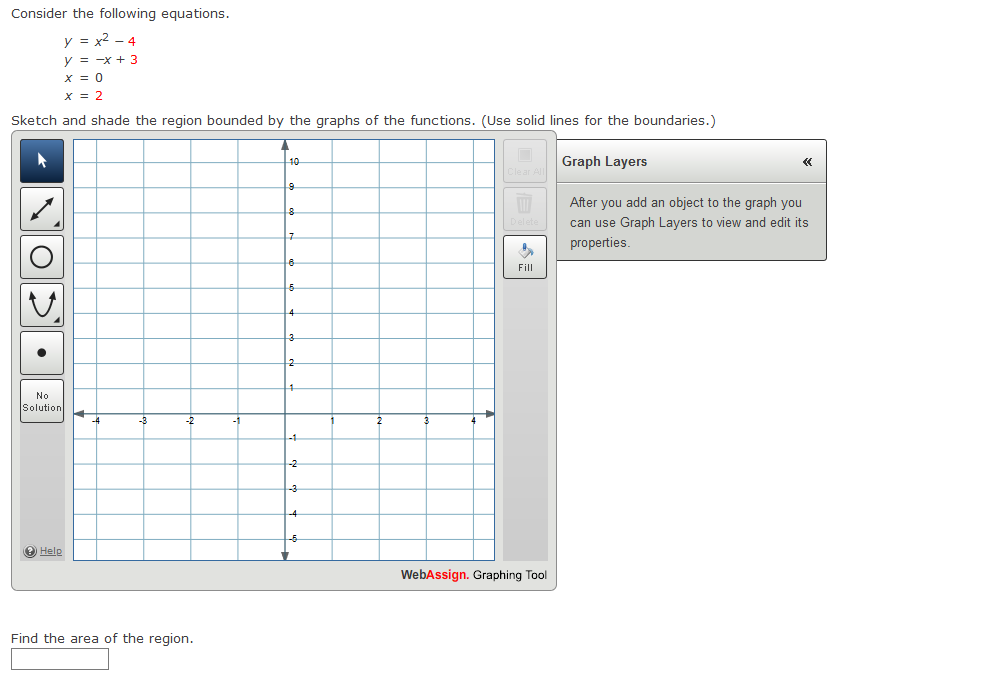



Solved Consider The Following Equations Y X2 4 Y X Chegg Com
Free PreAlgebra, Algebra, Trigonometry, Calculus, Geometry, Statistics and Chemistry calculators stepbystep(h,k) being vertex , here h = 2,k = 0,a = 1 Since a is positive, parabola opens upward Therefore vertex is at (h,k) or (2,0) Axis of symmetry is x = h or x = 2;3)y=x^24x •Graph this original function in its entire domain but coloring green that portion of the graph over the limited domain x>=2, •Find its inverse over x>=4, Inverse?
Enter {piecewisedefined function here the roots of this equation will be the inflection points for the specified function graph $$\frac{d^{2}}{d x^{2}} f{\left(x \right)} = $$ the second derivative $$8 = 0$$ Solve this equationGraph 4x2y=4 4x − 2y = −4 4 x 2 y = 4 Solve for y y Tap for more steps Subtract 4 x 4 x from both sides of the equation − 2 y = − 4 − 4 x 2 y = 4 4 x Divide each term by − 2 2 and simplify Tap for more steps Divide each term in − 2 y = − 4 − 4 x 2 y = 4 4 x by − 2 2Directrix y = −25 4 y = 25 4 Select a few x x values, and plug them into the equation to find the corresponding y y values The x x values should be selected around the vertex Tap for more steps Replace the variable x x with 1 1 in the expression f ( 1) = ( 1) 2 − 4 ⋅ 1 − 2 f ( 1) = ( 1) 2 4 ⋅ 1 2 Simplify the result
The graph of y=x^24x by drawing the line y=x4 UrMomIsSuperGay is waiting for your help Add your answer and earn points New questions in Mathematics The graph of y=x^24x by drawing the line y=x4 A hot air balloon is descending The height of the balloon n minutes after it starts to descend is hn metres The height of the balloon (n 1A Slope intercept form of the line is given by y=mxc, where m is slope and c is Yintercept Q y = 5 (x 2)2 4 6 Use the graphing tool to graph the equation Click to 2 enlarge graph 10 B Given Data The equation of the graph is 3x3y=9 The xGraph of y=4x^2 Answer Math Problem Solver Cymath \\"Get




How Do You Graph Quadratic Functions Y X 2 4x 7 Socratic



Question 2 The Diagram Shows The Graph Of Y X2 4x Gauthmath
Steps for Solving Linear Equation 4 y 4 x = 2 4 y 4 x = − 2 Subtract 4x from both sides Subtract 4 x from both sides 4y=24x 4 y = − 2 − 4 x The equation is in standard form The equation is in standard formPopular Problems Trigonometry Graph y^24x4=0 y2 − 4x − 4 = 0 y 2 4 x 4 = 0 Move all terms not containing x x to the right side of the equation Tap for more steps Subtract y 2 y 2 from both sides of the equation − 4 x − 4 = − y 2 4 x 4 =The graph from to Intersection points does show?



Solution Y X2 4x 7 What Is The Y Intercept X Intercept Turning Point Please Write Down The Solution As Well Thanks
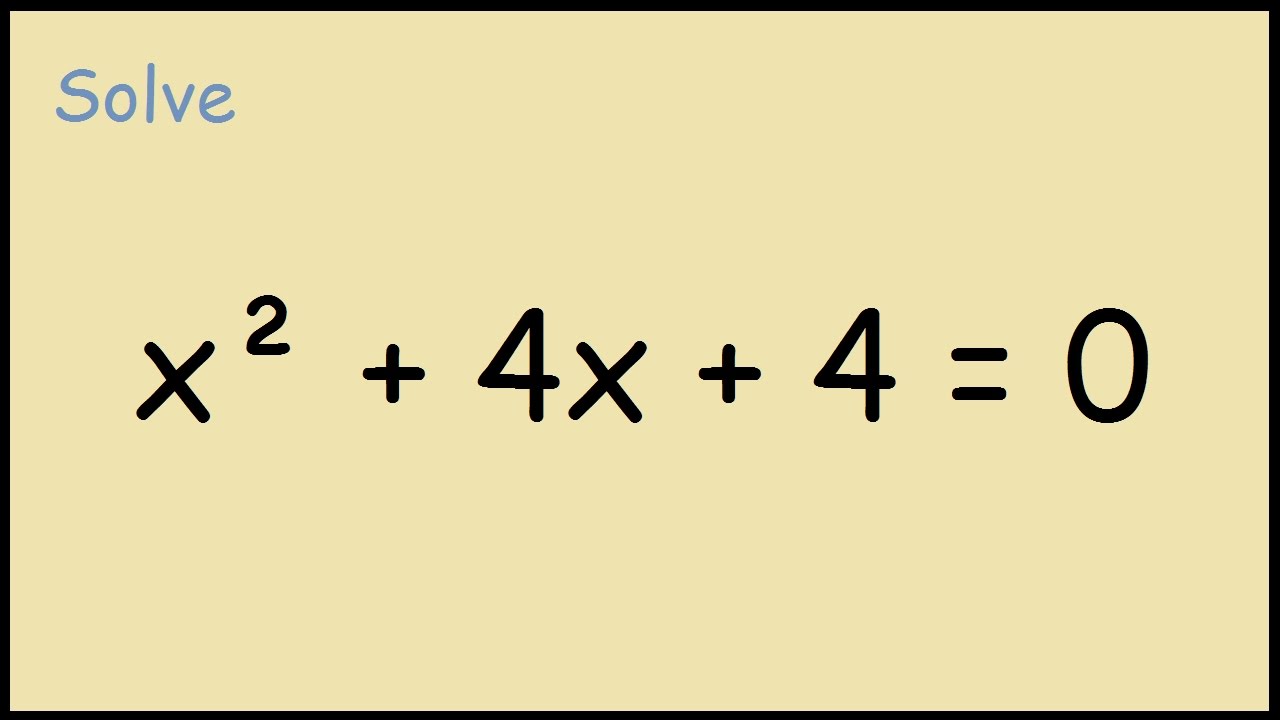



Solve X 2 4x 4 0 Youtube
Solution Given, the linear equations are x y 2 = 0 4x y 4 = 0 We have to draw the graph of the pair of linear equations and calculate the area of the triangle formed by the lines drawn and the xaxis The equation x y 2 = 0 can be written as y = x 2Equations y = x² 4·x and y = x 4 can be solved where they are equal as follows Thus equating the value This is a quadratic function of form y=ax^2bxc, with a=1, b=4 and c=5 The graph will be a parabola Since a is positive, the arms go up To find the yintercept, let x = 0 and find y = c = 5 To find the xintercepts, let y = 0 and use either factorizing or the quadratic formula to find the roots x = 5 or x = 1
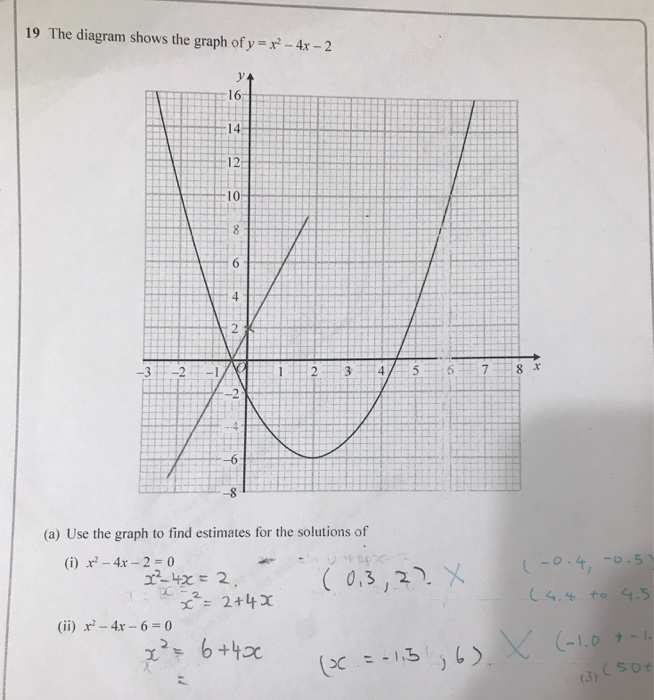



Solved 19 The Diagram Shows The Graph Ofy X2 4 2 16 12 10 Chegg Com




Graph Y 3 4 X 1 Youtube
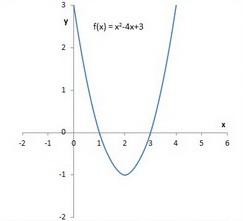
Find an answer to your question Complete the table of values for y=x^24x x = (1,0,1,2,3,4,5) please help me find y using the equation y=x(squared) 4x thank emcalleja emcalleja The yintercept of the line is at 0 What linear function is represented by the graph? First consider the equation y=x^24x This can be factored as y=x(x4) Note that this has zeros when y=0, which are when x=0 and x4=0=>x=4 The vertex of this parabola will be halfway between the zeros, at x=2 Note that y(2)=(2)^24(2)=4 the graph of y=x^2 4x 3 is shown The graph of is attached below From the graph we can see that , the vertex is (2,1) The graph changes direction at the point (2,1) so we can say that there is only one turning point at Now we find out the roots of the equation roots are the points where the graph crosses or touches x axis The




Solved Consider The Following Equations Y X2 4 Y X Chegg Com
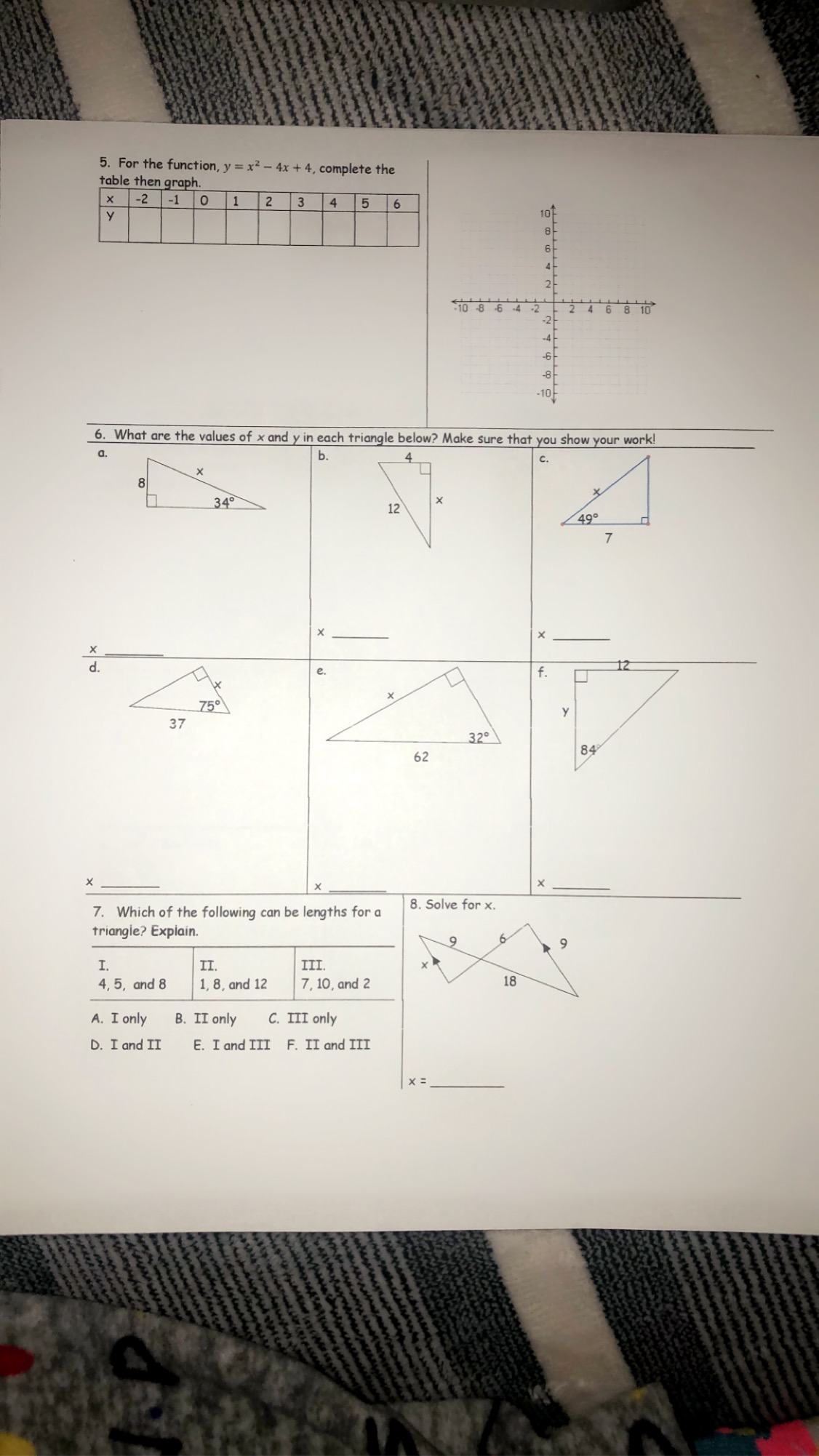



Answered 5 For The Function Y X 4x 4 Bartleby
The xintercept of the graph is the point where the graph cuts the xaxis So at that point, the y coordinate will be zero The given quadratic equation is y = x 2 4x 4 Taking y = 0 0 = x 2 4x 4 It is a perfect square of (x 2)Algebra Graph y= (x2) (x4) y = (x 2)(x − 4) y = ( x 2) ( x 4) Find the properties of the given parabola Tap for more steps Rewrite the equation in vertex form Tap for more steps Complete the square for ( x 2) ( x − 4) ( x 2) ( x 4) a) For 4x^24x 1 = 0 What we have to do here is to simply find he points on the xaxis in which the graph crosses it we have this as;
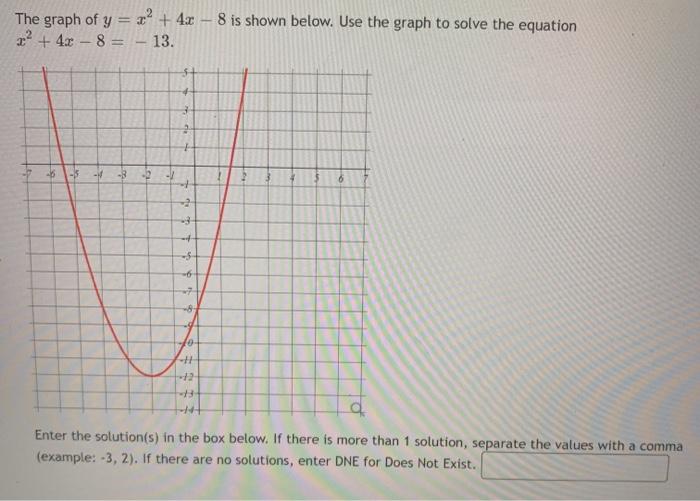



Solved The Graph Of Y X2 4x 8 Is Shown Below Use The Chegg Com




Which Of The Following Is The Graph Of The Quadratic Function Y X2 4x 4 Brainly Com
If x=2, then y=2 2−4×24=4−84=0 So, the points are (0,4),(1,1),(2,0) Plotting (0,4),(1,1),(2,0) and joining them Here, the graph cuts xaxis at one distinct point A The xcoordinates of A(2,0) is the one zeroes of the quadratic polynomial y=x 2− 4x4 Solve any question of Polynomials withY=x24x4 No solutions found Rearrange Rearrange the equation by subtracting what is to the right of the equal sign from both sides of the equation y(x^24*x4)=0 Step How do you graph the function, label the vertex, axis of symmetry, and xintercepts The solution of the system of equations, y = x² 4·x and y = x 4 is directly found from the graph by the points of the intersection of the lines y = x² 4·x and y = x 4 which are (4, 0) and (1, 3) x = 1 y = 3 or x = 4 y = 0 Stepbystep explanation To verify, we have;
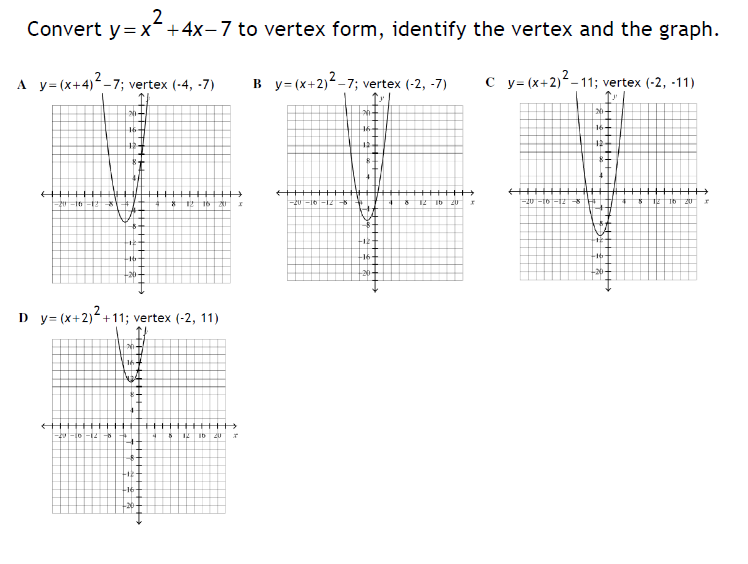



Solved 2 Convert Y X 4x 7 To Vertex Form Identify The Chegg Com



How To Solve Quadratic Equation By Factoring Video Tutorial Practice Problems Plus Worksheet With Answer Key
Explore math with our beautiful, free online graphing calculator Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and moreGraphing y = (4x4)*(x2) Function f() Plot the graph!Graph y^2=4x y2 = 4x y 2 = 4 x Rewrite the equation as 4x = y2 4 x = y 2 4x = y2 4 x = y 2 Divide each term by 4 4 and simplify Tap for more steps Divide each term in 4 x = y 2 4 x = y 2 by 4 4 4 x 4 = y 2 4 4 x 4 = y 2 4 Cancel the common factor of 4 4



Question 4 Edexcel Gcse Jun16 1h Q19b The Diag Gauthmath




The Graph Of Y X2 4x Is Shown On The Grid By Drawing The Line Y X 4 Solve The Equations Y Brainly Com
By finding values of y after assigning values to x If x is zero, y=4 If x is 1, y=3 If x is 2, y=0 and so on (it is a nonlinear function) The graph is provided below graph{(x^2) 4 909, 1091, 528, 472}Subtract y from both sides 4x^ {2}4x1y=0 4 x 2 − 4 x 1 − y = 0 This equation is in standard form ax^ {2}bxc=0 Substitute 4 for a, 4 for b, and 1y for c in the quadratic formula, \frac {b±\sqrt {b^ {2}4ac}} {2a} This equation is in standard form a x 2 b x c = 0Y=x^24x4 (graph 300x0 pixels, x from 6 to 5, y from 10 to 10, x^2 4x 4) Intercepts (0,4) (2,0) Proof using the (2,0) x & y values 0=2^24*24 0=484 0=0 Answer by ewatrrr (243) ( Show Source ) You can put this solution on YOUR website!




The Polynomial Y X 5 X 2 4x 3 Has Been Graphed Incorrectly Identify The Error And How Brainly Com




Graph The Following Quadratic Equation And State Their Nature Of Solution X2 4x 4 0 Mathematics Shaalaa Com
STEP 1 Find the line of symmetry Example y = 2x2 4x 1 ( ) 4 1 2 2 2 b x a = = = y x Thus theHow To Graph A Quadratic Equation 10 Steps With Pictures y = x2 y = ‑ x2 Wider than parent function, reflected in xaxis Given a parabola in Standard Form, y = ax2 bx c, • When a > 0, the parabola opens up • When a 0, the parabola x^24x4 = (x2)^2 0 vertex (2, 0) Graph D has vertex (2, 0) Advertisement Advertisement AlexTrusk AlexTrusk Answer look at x=0 and plug in 0 for any x you find in the equation f(0) = 0² 4*0 4 = 4 c is out of the race then let's do this again, but test for x=1 and x=2 f(1) = 1² 4*1 4 = 1If u=m due north and v=10m at 30⁰ e of n, find uv and




What Are The Vertex And X Intercepts Of The Graph Of Y X2 4x 21 Select One Answer For The Vertex Brainly Com



How Do You Graph F X X 2 4x 5 Socratic
Best answer 1 y2 = 4x is a parabola and y = x is a straight line passing through the origin 2 x2 = 4x ⇒ x2 – 4x = 0 ⇒ x (x – 4) = 0 ⇒ x = 0, 4 When x = 0, y = 0 and when x = 4, y =4 Therefore the points of intersection are (0, 0) and (4, 4) 3 Area bounded by the graphs = Area under the parabola in the first quadrant – AreaXintercept is found by putting y = 0 in the equation y = (x − 2)2 or (x − 2)2 = 0 or or x = 2 or (2,0) orApproximately05 and 15 what we are saying is that the plot crosses the xaxis at x = 025 and x = 125 ii) what we have to do here is to find the point at which y = 2



Exploration Of Parabolas



Solution Graph F X X 2 4x 3 Labeling The Y Intercept Vertex And Axis Of Symmetry
y 2 4 = x 2 4x 4 Now that the right hand side is a perfect square trinomial, factor it y 2 = (x 2) (x 2) y 2 = (x 2) 2 Subtract 2 from both sides y = (x 2) 2 2 When the equation is in this form, we can see that this is the graph of y = x 2 shifted to the right 2 units and shifted down 2 units The vertex is at (2, 2)See the entire solution process below Explanation Step 1) Subtract (4x) from each side of the equation to solve for y while keeping the equation More Items 4x=4y Subtract y from both sides \frac {4x} {4}=\frac {4y} {4} Divide both sides by 4 x=\frac {4y} {4} Dividing by 4 undoes the multiplication by 4The equation is now solved x^ {2}4x=y Swap sides so that all variable terms are on the left hand side x^ {2}4x\left (2\right)^ {2}=y\left (2\right)^ {2} Divide 4, the coefficient of the x term, by 2 to get 2 Then add the square of 2 to both sides of the equation This step makes the left hand side of the equation a perfect square




Slope Intercept Form Graph Y 5 4x 2 Youtube



How Do You Graph Y X 2 4x 21 Socratic
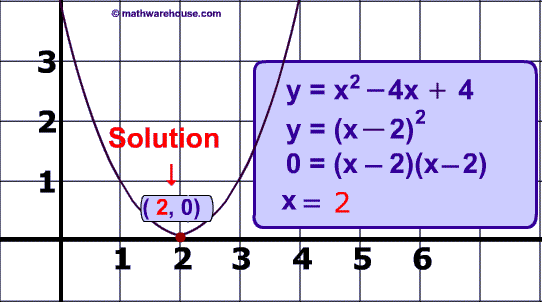
Free graphing calculator instantly graphs your math problems To graph y=2x−6 you would plot a point at (0,−6) at the yintercept, then go up 2 over 1 since the slope is 2 (same as 21 ) You would continue from that point going up 2 right 1 plotting points at each stop and then you can draw the lineGraph Quiz Polynomial 5 problems similar to x24x4 Final result (x 2)2 Step by step solution Step 1 Trying to factor by splitting the middle term 11 Factoring x24x4 The first term is, x2 its coefficient is 1 that the midpoint of r and s corresponds to the axis of symmetry of the parabola represented by the quadratic




What Is The Vertex Of F X X 2 4x 5 Socratic




Graph The Linear Equation Yx 2 1 Draw
Solution Verified by Toppr y=x 2−4x On factoring we get, x=0,x−4=0 The roots are x=0,4 Since the constant term yintercept is 0, this graph passes through the originAnswer (1 of 4) Question How do I graph this quadratic function y=x^24?Expert Answer Note that, f (x) = 2x2 4x 2 implies, f (x) = 2 (x2 2x) 2 ie f (x) = 2 (x2 2x 1) 2 2* (1) ie f (x) = 2 (x View the full answer Transcribed image text Graph the function f (x) = 2x² 4x − 2 by starting with the graph of y = x² and using transformations (shifting, stretching/compressing, and/or




Sonia Graphs The Equations Y X2 4x And Y X 4 To Solve The Equation X2 4x X 4 Her Brainly Com



How Do I Convert The Equation F X X 2 4x 3 To Vertex Form Socratic
Divide y by 1 x^ {2}4x2^ {2}=y2^ {2} Divide 4, the coefficient of the x term, by 2 to get 2 Then add the square of 2 to both sides of the equation This step makes the left hand side of the equation a perfect square x^ {2}4x4=y4 Square 2 We can easily graph the given function using translation properties of functions First, consider what the function y = x actually means It represents the absolute value, thus So then the graph of y = x − 2 is the same as the above graph except that it is translated right by 2 units Then finally the graph of y = x −2− 4 is the* Answer This is a great question because it helps us understand a very important aspect of Quadratic Equations and Parabolas As suggested by one other respondent, let's draw a



Solution Use The Graph Of Y X2 4x 5 To Answer The Following A Without Solving The Equation Or Factoring Determine The Solution S To The Equation 4 5 0 2 X Amp 614 Amp X
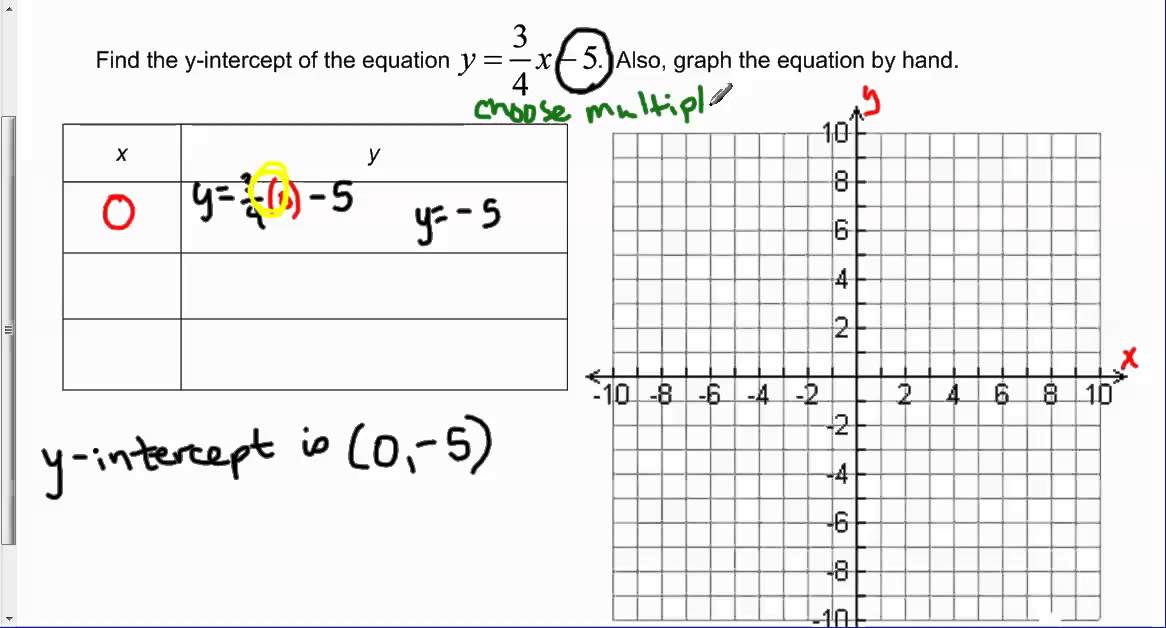



Find The Y Intercept Of Y 3 4x 5 And Graph Youtube
Showing how to graph a simple quadratic with 5 pointsSin (x)cos (y)=05 2x−3y=1 cos (x^2)=y (x−3) (x3)=y^2 y=x^2 If you don't include an equals sign, it will assume you mean " =0 " It has not been well tested, so have fun with it, but don't trust it If it gives you problems, let me know Note it may take a few seconds to finish, because it has to do lots of calculations c) The vertex is at (2,0) d) The parabola is dashed Stepbystep explanation We are given an algebraic inequality as y > x^2 4x 4 This implies that y> (x2)^2 The options that holds true regarding this inequality is a) The parabola opens up Since the coefficient of the leading term is positive c) The vertex is at (2,0) d) The parabola is dashed




Systems Of Equations With Graphing Article Khan Academy



Exploration Of Parabolas



A Complete The Table Of Values For Y X2 4x B Which Gauthmath
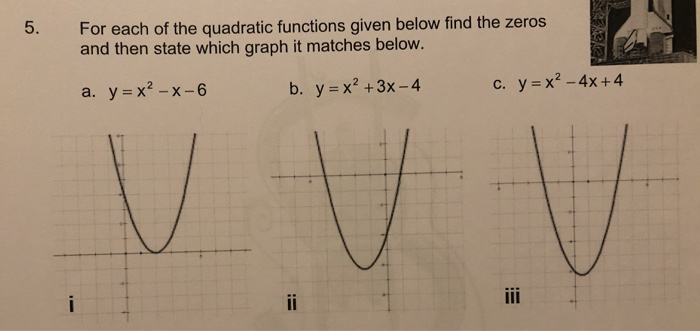



Solved 5 For Each Of The Quadratic Functions Given Below Chegg Com
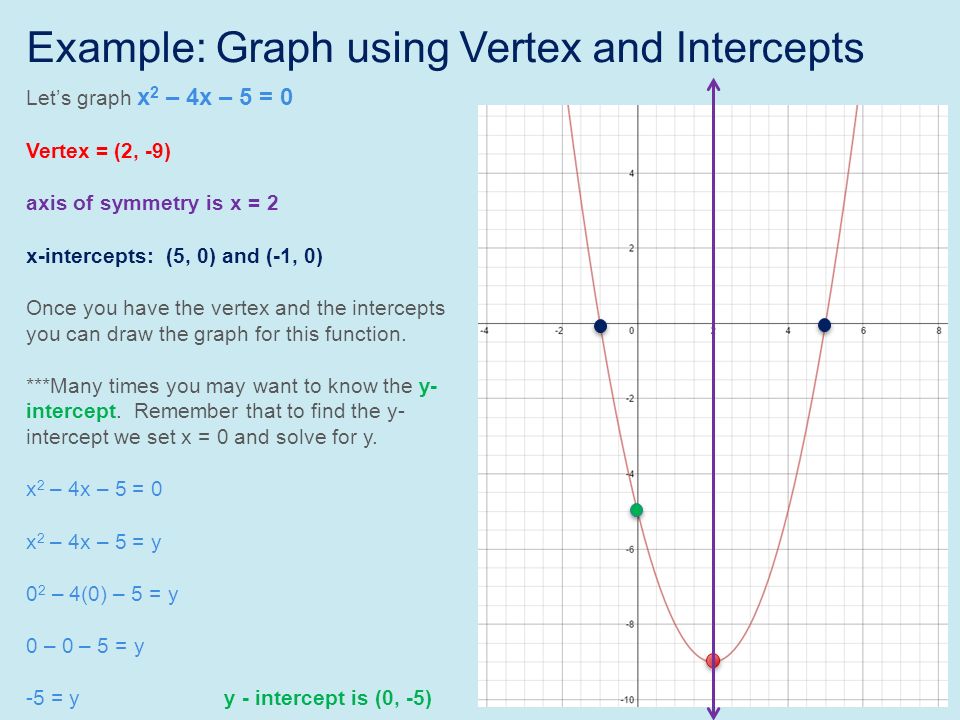



More Key Factors Of Polynomials Recall From Lesson 4 Standard Form Left To Right Factored Form The Fta Fundamental Theorem Of Algebra States That Ppt Download



Draw The Graph Of The Function Y X2 4x 6 Maths Topperlearning Com Knyzd6o11




Quadratic Graphs Parabolas Ppt Download




How To Sketch A Parabola Example 2 Y X 2 4x 12 Youtube



Parabolas
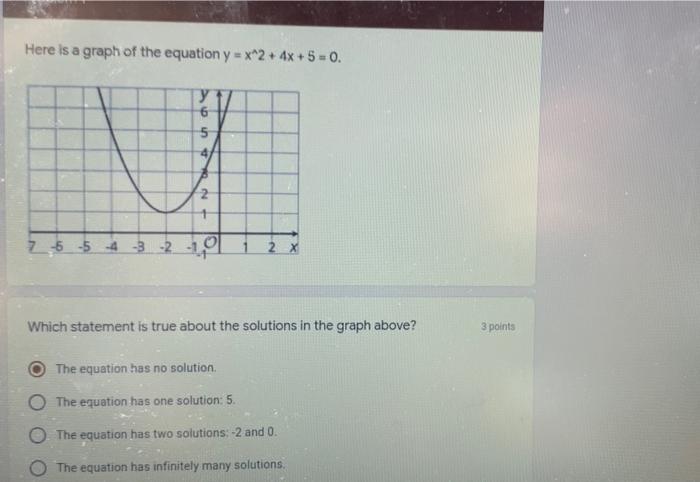



Solved Here Is A Graph Of The Equation Y X 2 4x 5 0 Chegg Com




Solved Nsider The Following Equation Y X2 4x 4 A Use Chegg Com




For The Curve Which Is The Graph Of The Equation Y X 4 4x 3 A Describe Where The Curve Is Increasing And Where Decreasing B Describe Where The Curve




Find The Area Of The Region Bounded By The Graphs Of Y X 2 4x And Y X 4 A 4 500 B 4 500 C 2 333 D None Of These Study Com




4 Which Of The Following Shows The Graph Of Y X2 4x 12 A B C D Brainly Ph
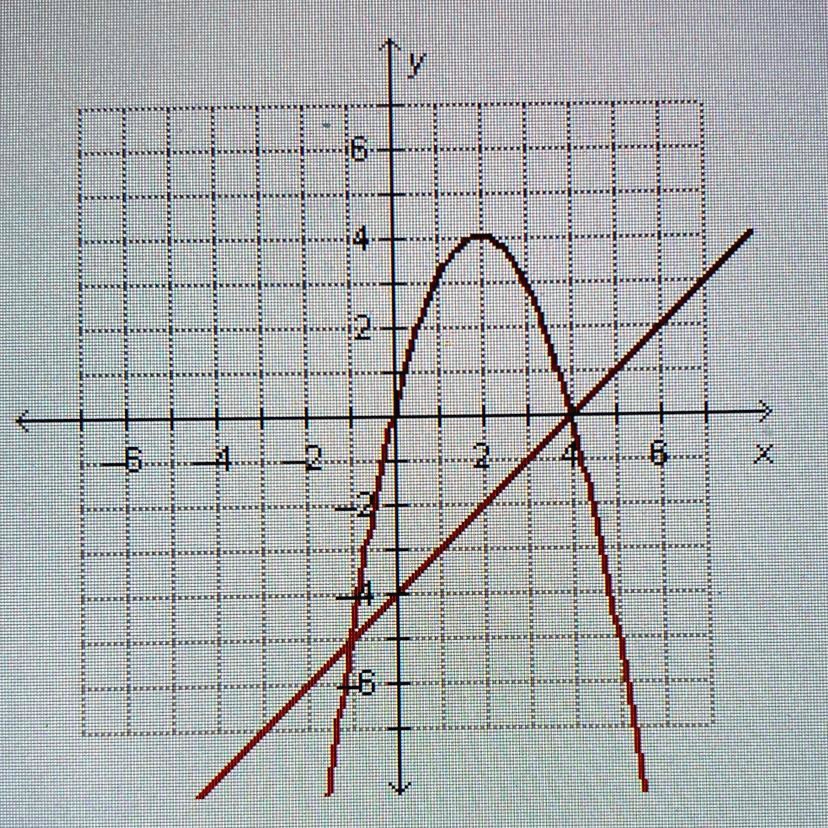



Solved Help Please Sonia Graphs The Equation Y X 2 4x And Y X 4 To Solve The Equation X 2 4x X 4 Her Graph Is Shown Below What Are The Solutions Of X 2 4x X 4 A 5 And 0 B 5 And 1 C 1




Plot The Graph For The Equation Y X 2 4x Sahay Sir
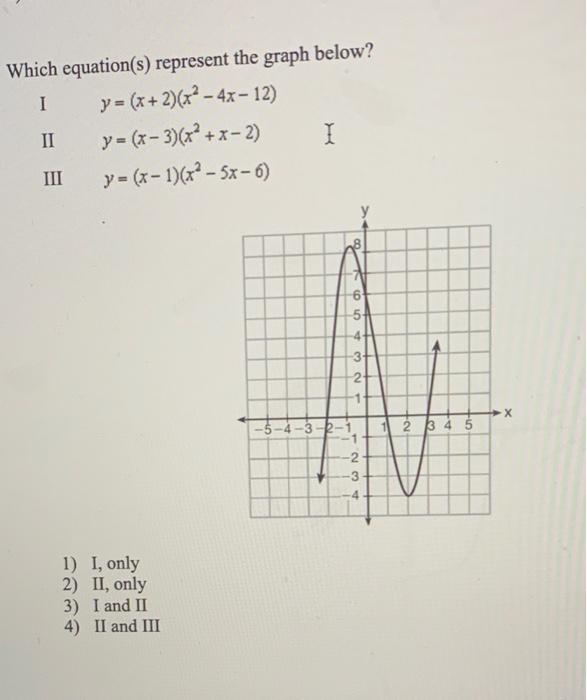



Solved Which Equation S Represent The Graph Below I Y X Chegg Com



26 Draw The Graph Of X 2 4x 4 Also Find Its Zeros Snapsolve
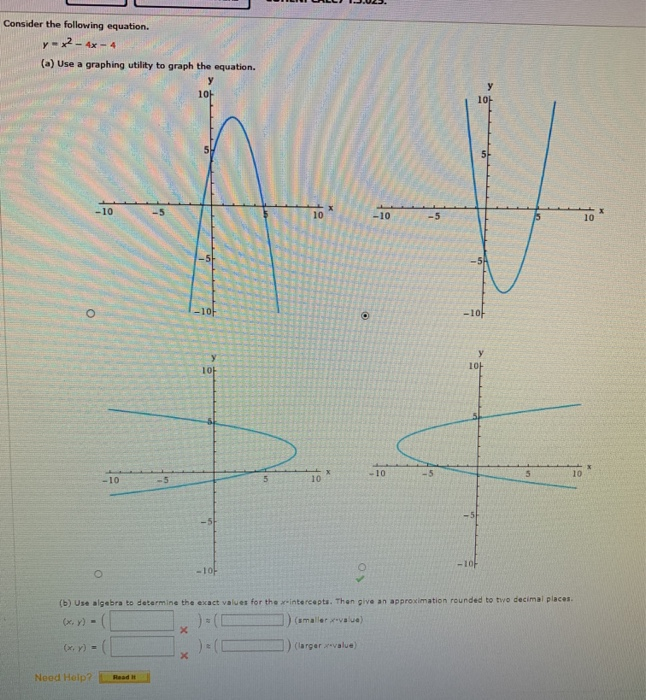



Solved Consider The Following Equation Y X2 4x 4 A Chegg Com



Draw The Graph Of Y X 2 3x 4 And Hence Use It To Solve X 2 3x 4 0 Y 2 X 3x 4 Sarthaks Econnect Largest Online Education Community



Graphing Quadratic Functions



What Is The Center Radius Form For The Equation X 2 Y 2 4x 6y 4 0 And Graph The Circle On The Cartesian Plane Quora
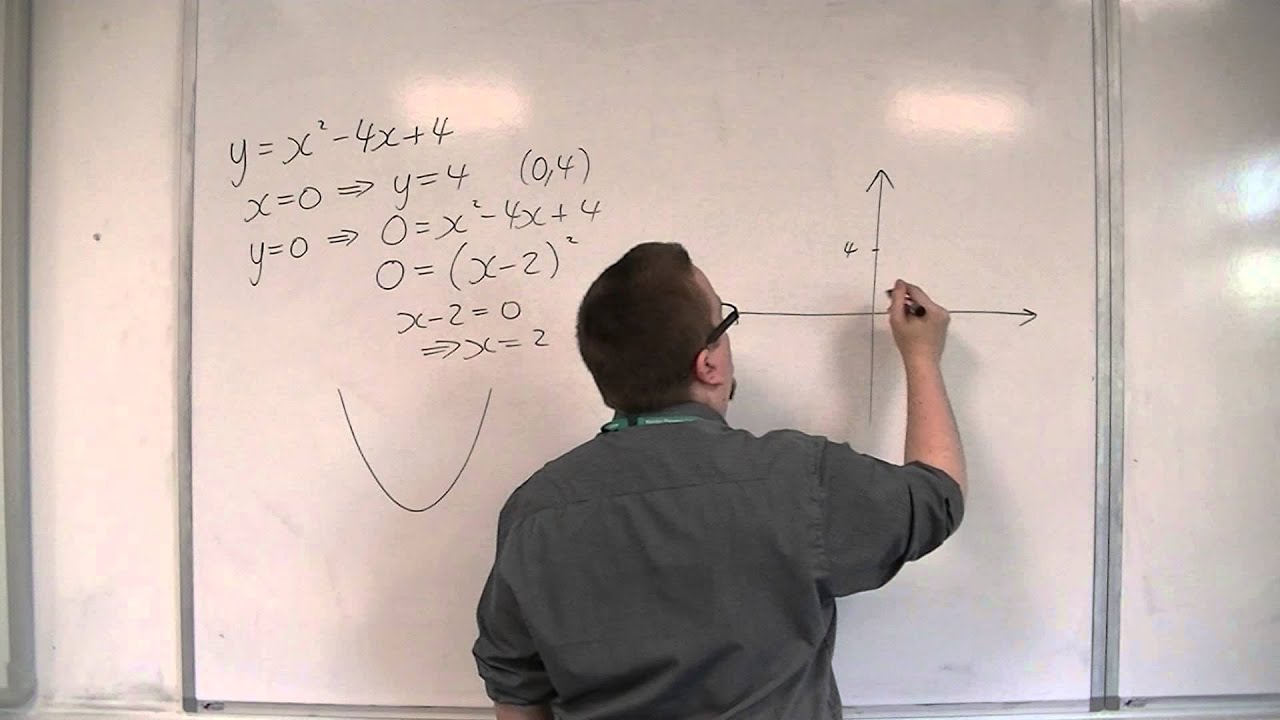



Ocr Mei Core 1 3 04 Sketch The Graph Of Y X 2 4x 4 Youtube
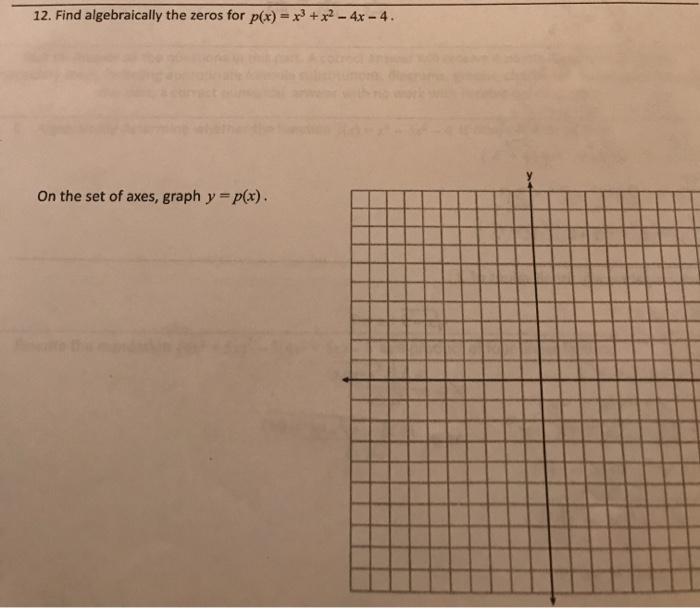



Solved 12 Find Algebraically The Zeros For P X X3 X2 Chegg Com



X2 4x 5 0



The Diagram Shows The Graph Of Y X2 4x 2 Use The G Gauthmath



Solution Question Sketch A Graph Of The Function Y 4x 4 6x 3 6x 2 4x Thank You



Image The Graph Of Equation Y X 2 4x 3 Equation
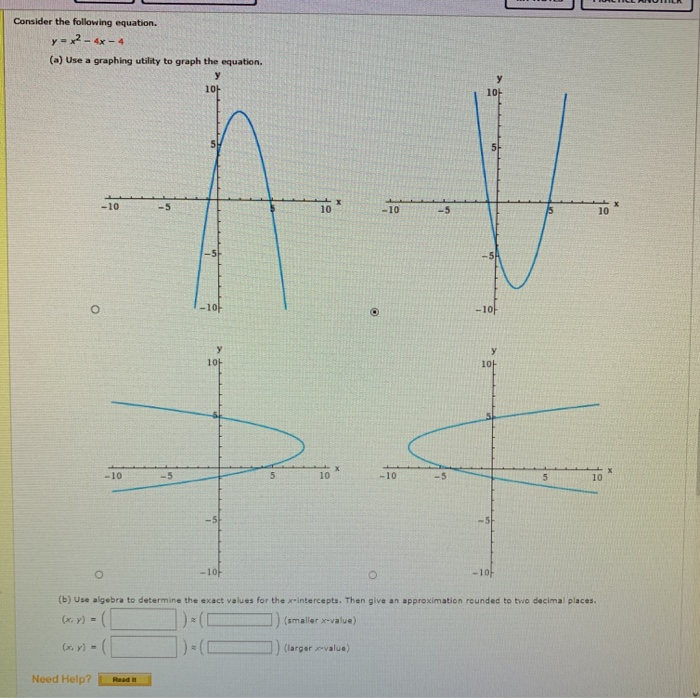



Solved Consider The Following Equation Y X2 4x 4 A Chegg Com



Solution Can You Pls Help Me 3 Y X 2 4x Graph This Original Function In Its Entire Domain But Coloring Green That Portion Of The Graph Over The Limited Domain X Gt 2 Find Its




The Figure Shows The Graph Of Y X2 4x C



Solution Draw The Graph Of Y 4x X2 For Values Of X For Which 2 Lt X Lt Find The Root Of The Equation



Plot A Graph For The Equation Y X 2 4x 1 Sarthaks Econnect Largest Online Education Community



Quadratic Graphs 1 A Complete The Table For Y X2




Sketch The Graph Of Y X 4x 5 Brainly Ph



Sketch A Graph Of The Circle Described By The Equation X 2 4x Y 2 6y 3 Quora



Graph Y X 7 X 3 Mathskey Com




Click On All That Are True About The Graph Of Y Gt X 2 4x 4 A The Parabola Opens Up B The Brainly Com
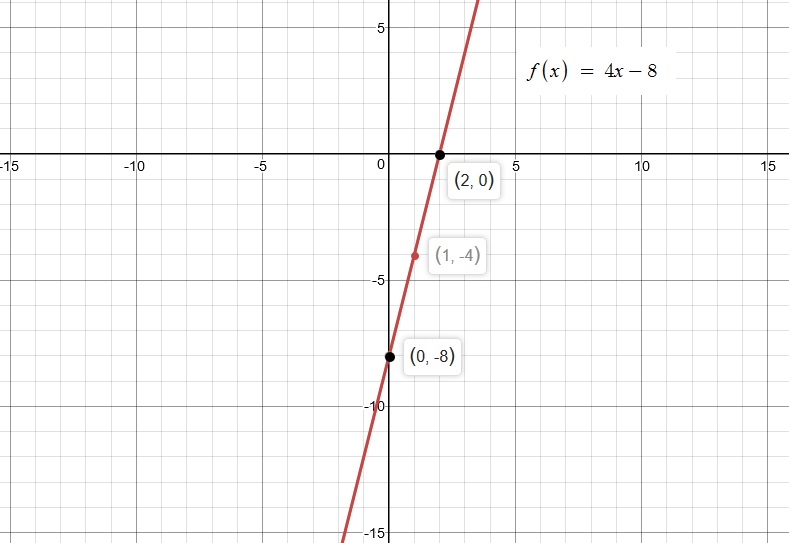



How Do You Graph Y 4x 8 Socratic
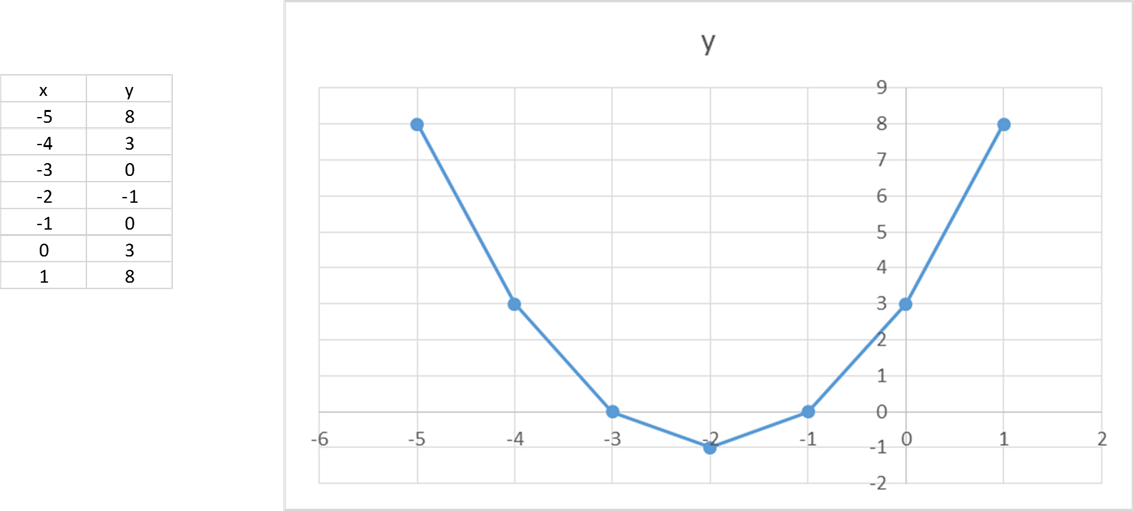



How Do You Graph Y X 2 4x 3 Socratic
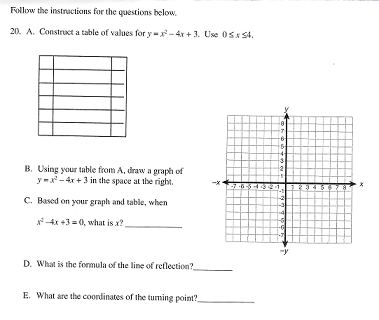



Solved Follow The Instructions For The Questions Below Chegg Com
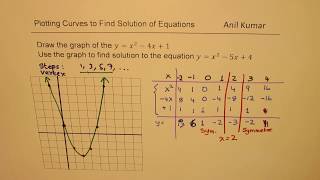



Step To Draw Y X 2 4x 1and Find Solution To Quadratic Equation Y X 2 5x 4 Youtube




Find The Area Of Y X2 4x Y 2x Study Com



Where Do Y X 2 4x 1 And Y 3 X Intersect Mathskey Com



The Graph Of Y X2 4x Is Shown On The Grid Y By Dr Gauthmath



Y X2 4x 3




Quadratic Polynomial Y X 2 4x 4 Draw A Graph And Represent The Zeroes Of The Polynomial
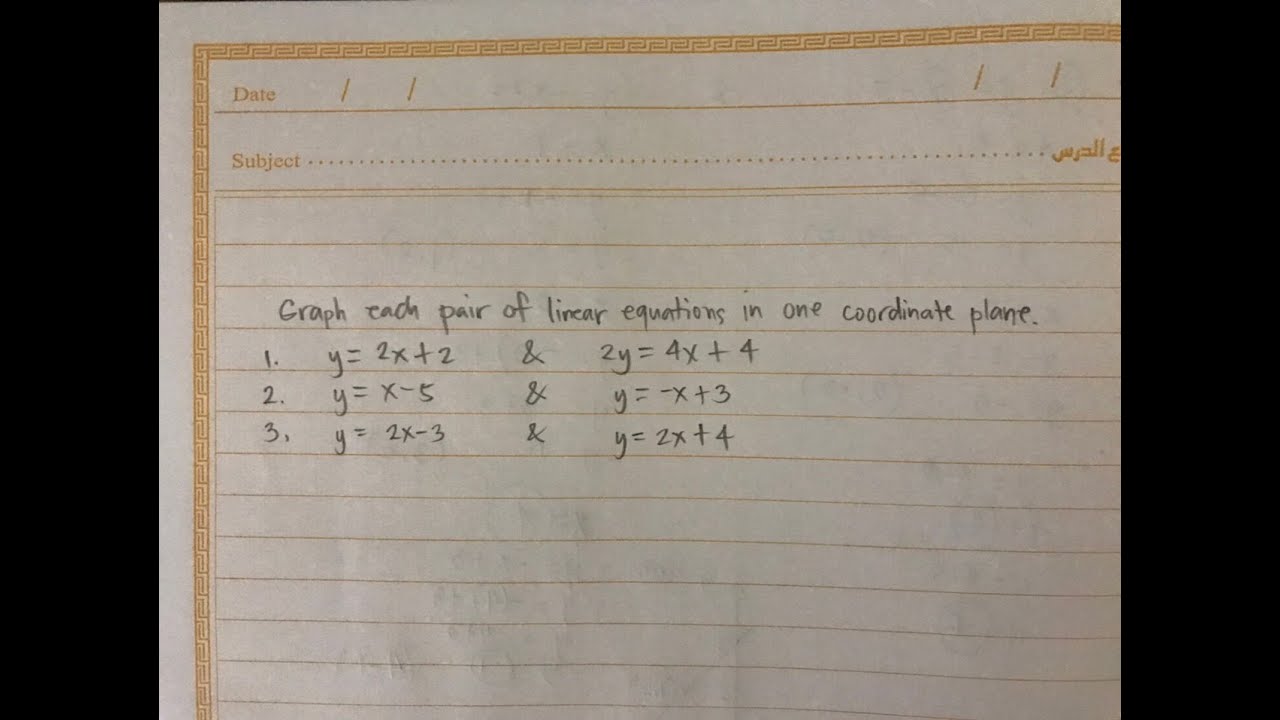



Graph Each Pair Of Linear Equations In One Coordinate Plane Youtube




The Graph Of Y X 2 4x 3 Is Shown Work Out A And B Please Brainly Com



How To Graph Y 4x 2 Quora



Question 2 The Diagram Shows The Graph Of Y X2 4x Gauthmath



Solution 2 For The Function Y X2 4x 5 Perform The Following Tasks A Put The Function In The Form Y A X H 2 K Answer Show
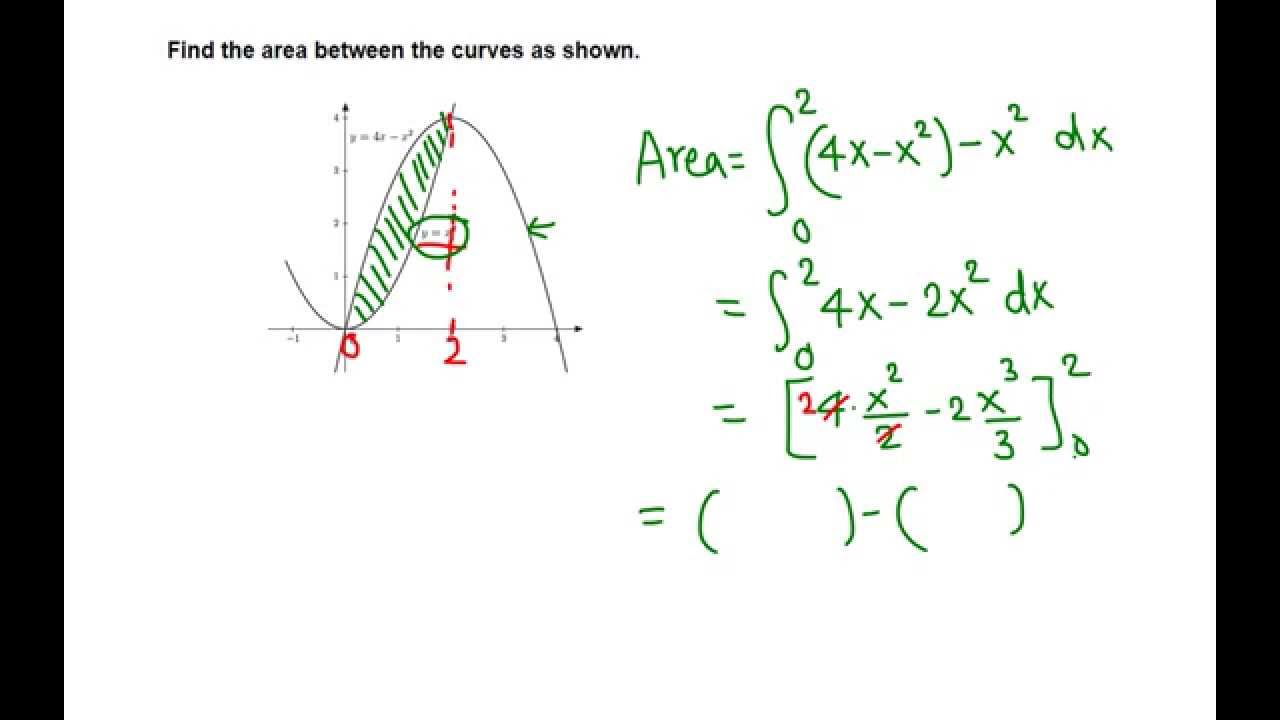



Area Between Y 4x X 2 And Y X 2 Youtube



1



Graph The Parabola Y X 2 4x 1 Youtube



Graphing Quadratic Functions




How Do You Solve By Graphing Y 4x 4 And 3x 2y 12 Socratic




The Graph Of Y X2 4x Is Shown On The Grid Find The Range Of Values For Which X 4x Lt 0 Brainly Com
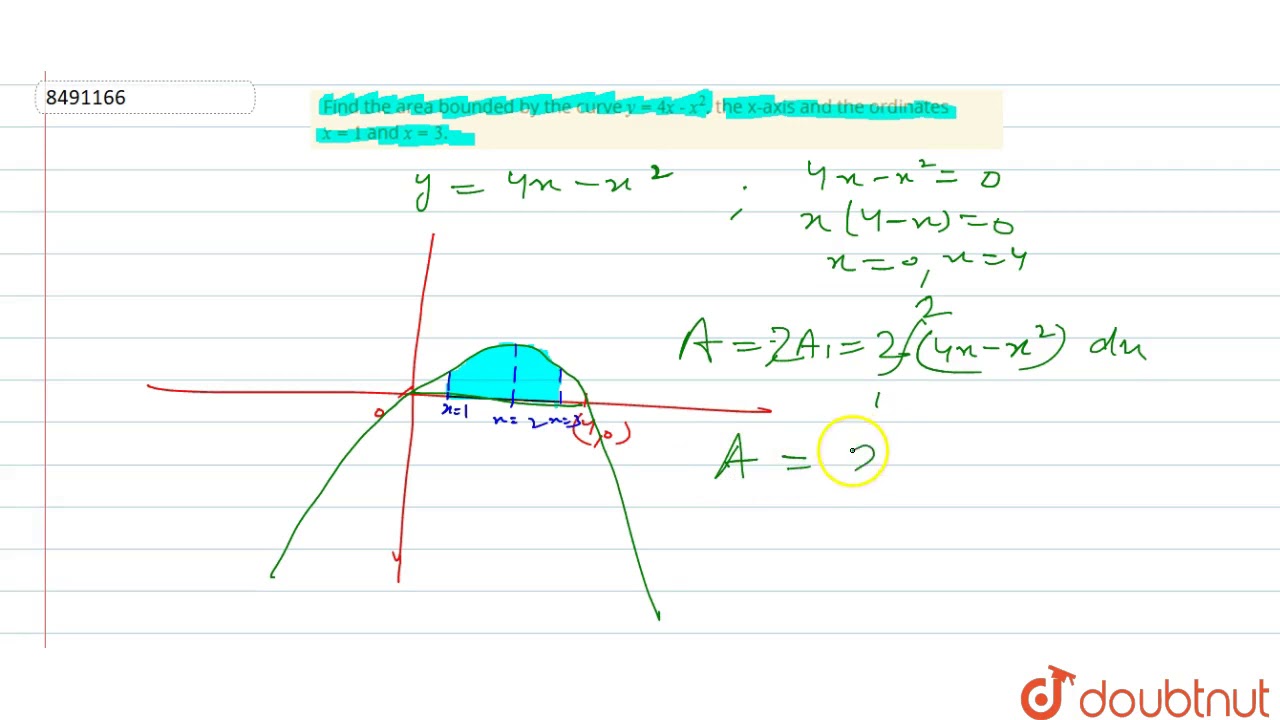



Find The Area Bounded By The Curve Y 4x X 2 The X Axis And The Ordinates X 1 And X 3 Youtube



Solution Graph The Parabola Y X2 4x 4 Use The Quadratic Formula To Solve The Equation To The Exact Value Or Round To Two Decimal Places 2x 2 X 1



Pplato Basic Mathematics Quadratic Functions And Their Graphs
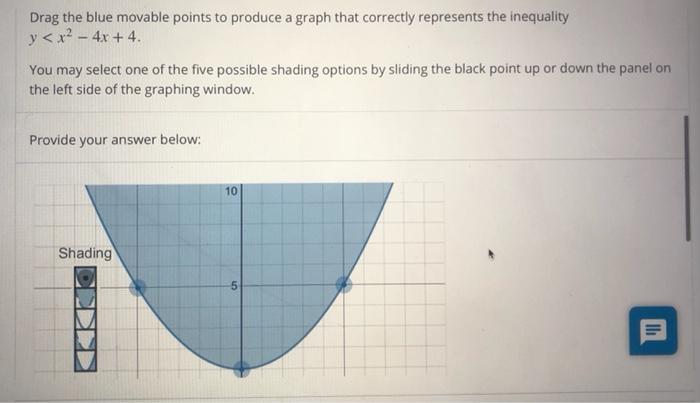



Solved Drag The Blue Movable Points To Produce A Graph That Chegg Com



Solution What Are The Intercepts For The Equation Y X 2 4x 4



Solution Graph Y 2x 2 4x
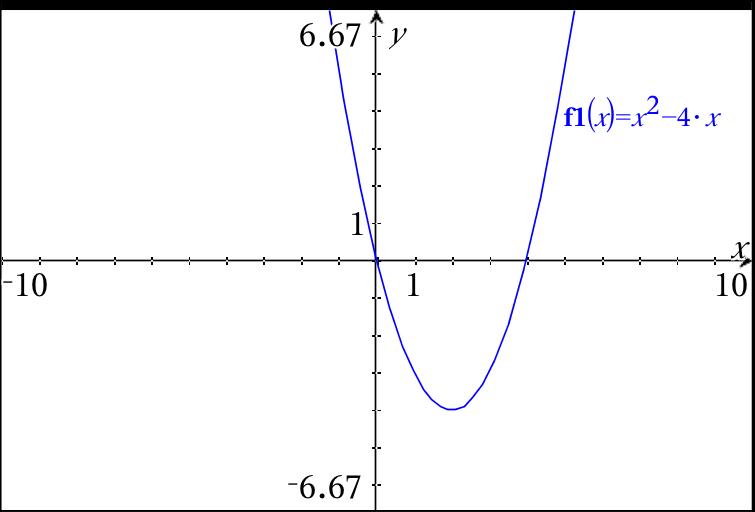



What Is The Graph Of F X X 2 4x Socratic




Which Of The Following Is The Graph Of The Quadratic Function Y X 2 4x 4 Brainly Com




Solved Complete The Table Using Y X2 4x 2 Then Plot Chegg Com



A Transform The Given Quadratic Functions Into Th Gauthmath
コメント
コメントを投稿